Shared Publication

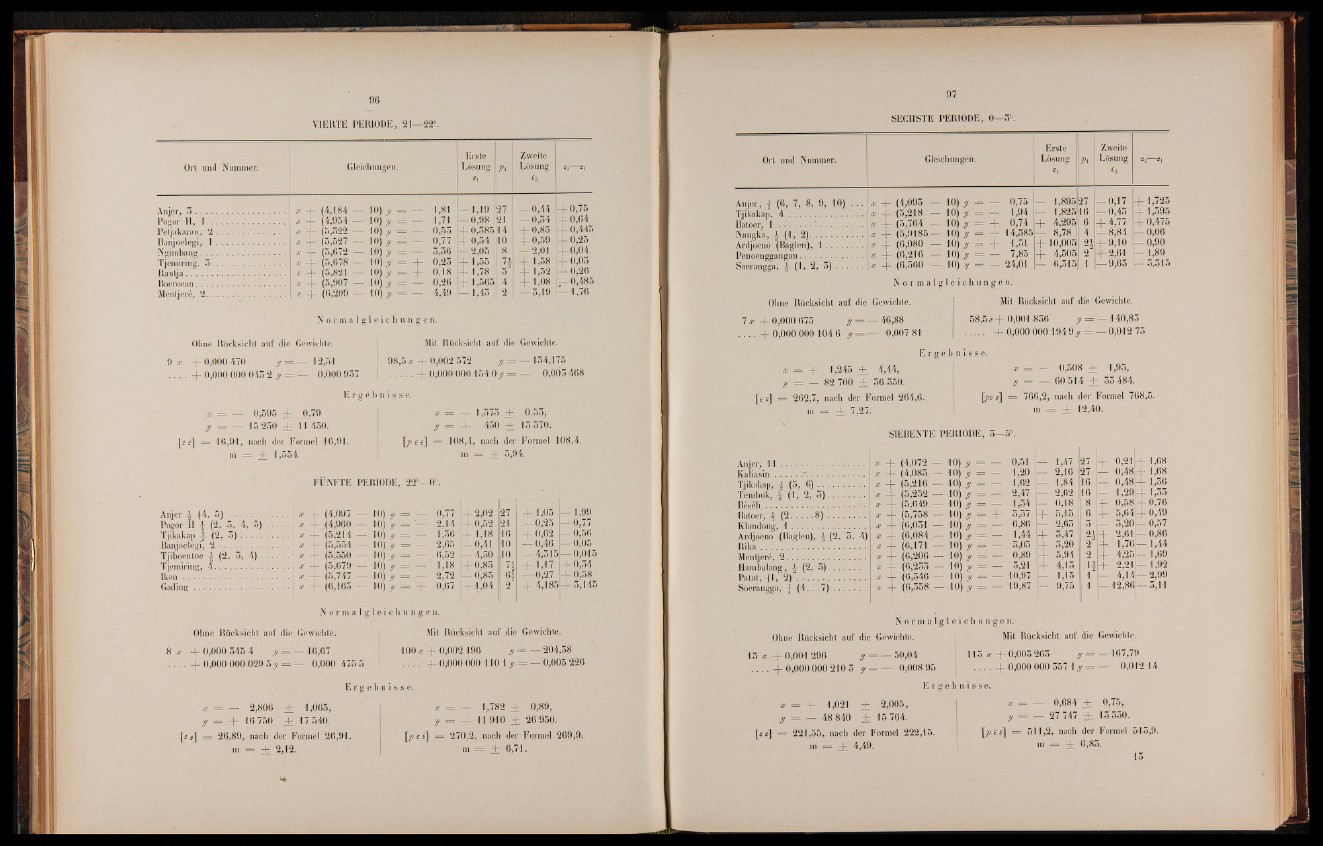
96 VIERTE PERIODE, 21— 22h. Ort und Nummer. Gleichungen. Erste 1 Lösung £| V Zweite Lösung $2 Ê2---fl Anjer, 5 ........................................ a? + (4,1,84 S io) i B l l 1,81 M 1,19 27 — 0,44 § 0 , 7 5 Pogor II, 1 ................................. x + (4,954 — 10) y - m i 1,71 S -0 ,9 8 21 — 0,34 m 0,64 Petjakaran, 2 ............................... x + (5,522 +> lo i S a l 0,55 9 0,38514 + 0,83 Ü 0,445 Banjoelegi, ............................... x + (5.527 — 10) y B 0,77 # 0 , 3 4 10 4 - 0,59 + 0,25 Ngimbang...................................... x + (5,672 — 10) 1 = - 3,56 5 2.05 8 5 ~ -2,01 ü 1,58 + 0,04 Tjemiring. 5 ................................. x + (5,678 — i o » . K 9 0,23 9 1 , 5 5 71 B i Rantja............................................. a? + (5,821 - 10) y 9 9 0,18 4 -1 ,7 8 | 5 äM-1,52 — 0,26 à? + (5,907 — ■ i MË 10)y = - l é i c h u n g e 0,26 4,49 n. + 1,565 4 — 1,45 2 4 - 1,08 fem 4 8 5 MentjerÖ, 2. . . . , . .■-¡,................... x + (6,209 — N o r m a I g 5,19 — 1,76 Ohne Rücksicht auf die Gewichte. 9 « + 0 ,0 0 0 470 y = — *2,51 . . , . + 0,000 000 045 2 y = 'M 0,000 957 Mit Rücksicht auf die Gewichte. 98,5 x + 0,002 572 ¿N |f— 154,175 . . - . . . + 0,000 000 154 0 y 4 8 - 0,005 468 Er g e b n i s s e . x = — 0,595 + + 0 , 7 9 y .==•• -— 15 250 + 11 450. [ f i] = 16,91, nach der Formel 16,91. nV+M. • + 1,554. a?|l|i|j9g 1,575- + 0.55, y -.==• + 450 + 15 570. [p e e] = 108,4, nach der Formel 108,4. m = + 5,94. FÜNFTE PERIODE, 22h—Oh. Anjer £ (4, 5) . . . . . . . Pogor II \ (2, 5, 4, 5) Tjikakap % (2, 5 ) ......... Banjöe'legi, 2 ................... Tjiboenloe % (2, 5, 4) . Tjemiring, 4 ..................... Ik an ; .......... Gading............................... x }.- (4,097 - 10) y = - 0,77 fc|- 2,02 27 + 1,05 - x -f- (4,960 — io ) § a | 2,14 + 0,52 21 5 0.25 - « ■ ( 5 ,2 1 - 4 - 10) y = 5 1,36 f-)-l,1 8 1 9 m - 0,62 1 x jO-' (5,5o4 — 10) y H 2,65 — 0,41 10 — 0,46 - x +■ (5,550 ■ io). 6,52 I I . 4,50 10 — 4,315 - x j (5,679 — 10) y = ^ 1,18 $4- 0,83 n \ a 1,17 - x (5,747 — 10) B 2,72 — 0,85 ;4r- 0,27 - X + (6,165 t— 10) S . . v | 0’67 9 1,04 2 - f 4,185 - No rma l Ohne Rücksicht auf die Gewichte. + 0,000 545 4 y-- + 0,000 000 029 5 y -- 16,67 0,000 475 5 1,99 -0,77 -0,56 -0,05 [ 1 e-i ch u n g e n. Mit Rücksicht auf die Gewichte. 100 a?+ 0 ,0 0 2 196 y = — 204,58 . ; . . + 0,000 000 110 1 y = — 0,005 226 Er g e b n i s s e . x = — 2,806 + 1,065, y = + 16 750 + 17 540. [e e\ = 26,89, nach der Formel 26,91. in = §9 2 ,1 2 . x '= 4 M 1,782 + 0,89, y = 11 910 ± 26 950. [p e e] = 270,2, nach der Formel 269,9. m = , + 6,71. SECHSTE PERIODE, 0—5 \ Ort und Nummer. Gleichungen. Erste Lösung et Pt Zweite Lösung ei.-.ü s2—e, Anjer, V (6, 7, 8, 9, 10) . . . '®§ft;';i4.095 — 10) v = — 0,75 1 1,895 27 — 0,17 + 1,725 Tjikakap, 4 .......................... (5,218 9 10) y ■ — 1,94 5 1,825 16 — 0,43 + l,o95 Batoer, 1 ..............* ..................... ® ; + (5,764 9 10) y = + 0,74 + 4,295 6 + 4,77 S 0,475 Nangka, i (1. 2). . . .............. H + (5.9185— 101 v = — 14,585 — 8,78 4 — 8,84 — 0,06 Ardjoeno (Baglen), 1 ................ m + (6,080 # 10) y I f l s : 1,31 + 10,005 2 ) + 9,10 1 0 , 9 0 Penoenggangan............................ » M . (6-216 9 101% « ^ 9 7.85 -(- 4,505 2 + 2,61 9 . l',89 Soerangga, 4 (1, 2, 3) ............ x + (6,560 — 10) y = — 24,01 — 6,515 1 — 9,63 — 5,515 Oline Rücksicht auf die Gewichte. 7 x + 0,000 675 y = % 46,88 3 B B 0,000 000 104 6 y = — 0,007 81 E r j x 1,245 + , : 4,44, y pp — 82 700 “+ 56 550. 262,7, nach der Formel 264,6. m = £§ 7,27. N o rma l g l e i c hung e , n. Mit Rücksicht auf die Gewichte. 58,5a?+ 0,001 856 y = + 140,85 . . . + 0,000 000 194 9 y = ;4 - 0,012 75 e b n i s s e. 0,508 ' + g l , 9 5 , y = -— 60 514 + 55 484. [pee] = 766,2, nach der Formel 768,5. m = + 12,40. 1 SIEBENTE PERIODE, 5—5 \ Anjer, 1 1 ..................... Kaiiasin.......... Tjikakap, \ (5, 6) . . . Tembok, £ (1, 2, 5 ). Bèsèh . Batoer, \ (2 Klandong, 1 .......... Ardjoeno (Baglen), Rika........................ Mentjeréy 2 ............ Hambalang, ^ (2, Palat, (1, 2) . . . . Soerangga, £ (4. . 8 ) . . H M m X » (4,072 ■ x + (4,085 • x + (5,216 x + (5,252 • x + (5,649 x ~[- (5,/58 x + (6,051 — 10) y x + (6,084 a? + (6,171 x + (6,206 x + (6,255 x + (6,546 x + (6,558 10) y S 4 0 ) , = 1 0 ) , B mi o ) ,m 9 i o ) , a 1 0 ) , 1 0 ) , ■ 0,51 __ 1,47 27 + . 0,2-1 + 1,68 ■ 1,20 ■ 2,16 27 0,48 + 1,68 1,62 ■ 1,84 16 — 0,48 + 1,56 i— 2,47 — 2,62 16 9 1,29 + 1,55 ■ 1,54 ■ 0,18 8 s 0,58 + 0,76 3,57 + - 5,15 6 9 5,64 + 0,49 6,86 2,65 3 s 3 ,2 0—ä 0,57 1,44 9 5,47 9 + 2,61 — 0,86 H 3,03 + 3,20 2 “ 9 1/76 — 1,44 - - - 0,89 + 5,94 2 9 4 ,2 5—! 1,69 5,21 9 4,13 11 2,2 1—| 1,92 ■ 10,97 1,15 1 4,14 — 2,99 ■ 19,87 9 9,75 1 9 12,86—g 5,11 II N o r ma l g l e i c h u n g e n . Ohne Rücksicht auf die Gewichte. 13 x + 0,001 296 . , W — 50>04 . . . . + 0,000 000 210 3 , = — 0,008 95 | • . ’ , E ]■ g e b n i s s e. Mit Rücksicht auf die Gewichte. 113 x -(-0,003 265 167,79 . . . 4 - 0,000 000 357 1 §9 0,012 14 1,021 48 840 j g j | 2,005, H 15 764. [r £]!?§= 221,55, nach der Formel 222,15. m M + 4,49. k . ? " 0,684 + 0 , 7 5 , 27 747 4)1113 550. = 511,2, nach der Formel 515,9. m jB flM 6,85.